[경고] 아래 글을 읽지 않고 "라플라스 변환의 성질"을 보면 바보로 느껴질 수 있습니다.

[그림 1] 교류 회로 해석에 이용된 라플라스 변환(출처: wikipedia.org)
푸리에 변환(Fourier transform)의 복소 영역(complex domain) 일반화인 라플라스 변환(Laplace transform)의 다양한 성질은 푸리에 변환의 성질을 기반으로 쉽게 유도될 수 있다.
1. 기본(basics)
[정의]
 (1.1)
(1.1) (1.2)
(1.2)[선형 사상(linear mapping or linearity)]
 (1.3)
(1.3)여기서
[시간 및 주파수 편이(time and frequency shifting)]
 (1.3)
(1.3)[증명]
식 (1.1)에 시간 이동된 함수를 대입한다.
 (1.4)
(1.4)주파수 이동도 식 (1.4)와 비슷하게 적분할 수 있다.
______________________________
[시간 비율 조정(time scaling)]
 (1.5)
(1.5)
 (1.6)
(1.6)
 (1.7:
(1.7:
 (1.8:
(1.8:
 (1.9)
(1.9)
 (1.10)
(1.10)
 (1.11)
(1.11)
 (1.12)
(1.12)
 (1.13)
(1.13)
결국 의심스러운 부분 없이 명확하게 식 (1.9)의 첫째식이 불연속의 경우에도 잘 성립함을 알 수 있다.
 (1.14)
(1.14)
 (1.15)
(1.15)
 (1.16)
(1.16)
여기서 라플라스 변환은 명확한 식 (1.11)을 사용하며
 (1.17)
(1.17)
 (1.18)
(1.18)
 (1.19)
(1.19)
따라서 불연속 함수의 경우에도 식 (1.17)이 잘 성립한다.
 (1.20)
(1.20)
 (1.21)
(1.21)

 (1.22)
(1.22)
여기서
 (1.23)
(1.23)
 (1.24)
(1.24)
 (2.1)
(2.1)
 (2.2)
(2.2)
여기서
 (3.4)
(3.4)
 (3.5)
(3.5)
 (3.6)
(3.6)

 (4.5)
(4.5)
여기서 지수 함수의 수렴을 위해
 (4.6)
(4.6)
식 (4.6)을 이용해 식 (4.5)를 정리하면,
 (4.7)
(4.7)
 (4.8)
(4.8)
함수
 (4.9)
(4.9)
예를 들어,
 (4.10)
(4.10)
여기서

 (4.11)
(4.11)
 (1.5)
(1.5)여기서
[증명]
변수
 (1.6)
(1.6)______________________________
[켤레 복소수(complex conjugate)와 대칭성(symmetry)]
 (1.7:
(1.7:  (1.8:
(1.8: 여기서
[미분(differentiation)]
 (1.9)
(1.9)여기서
[증명]
라플라스 변환의 정의에 대해 부분 적분(integration by parts)을 적용한다.
 (1.10)
(1.10)여기서
______________________________
식 (1.9)에서 특이한 점은
 (1.11)
(1.11)예를 들어, 식 (1.1) 대신 식 (1.11)을 사용하면,
새로운 정의인 식 (1.11)의 라플라스 변환을 쓰면, 적분은
 (1.12)
(1.12) (1.13)
(1.13)[적분(integration)]
 (1.14)
(1.14)[증명]
식 (1.14)에 나온 적분을 식 (4.1)의 길쌈(convolution)으로 바꾸어서 식 (4.3)과 같은 라플라스 변환을 적용한다.
 (1.15)
(1.15)______________________________
[특수한 변환값]
 (1.16)
(1.16)[초기값 정리(initial value theorem)]
 (1.17)
(1.17)[증명]
식 (1.1)에
 (1.18)
(1.18)식 (1.18)의 최종 결과에
 (1.19)
(1.19)______________________________
미분의 라플라스 변환인 식 (1.9)의 첫째식과 식 (1.17)을 비교하면
[최종값 정리(final value theorem)]
 (1.20)
(1.20)[증명]
식 (1.19)의 마지막식에
 (1.21)
(1.21)그러면 식 (1.21)이 쉽게 증명되지만 조심할 부분도 있다. 극한

[그림 1.1] 라플라스 역변환을 위한 닫힌 경로
라플라스 변환
______________________________
최종값 정리와 다르게 식 (1.17)에 있는 초기값 정리는
[주기 함수(periodic function)]
 (1.22)
(1.22)[증명]
주기 함수의 특성인
 (1.23)
(1.23)______________________________
[거듭제곱 함수(power function)]
 (1.24)
(1.24)여기서
[증명]
라플라스 함수의 정의인 식 (1.1)을
______________________________
단순한 거듭제곱 함수
2. 초등 함수의 변환(transform of elementary functions)
[단위 계단 함수(unit step function)]
 (2.1)
(2.1)여기서
[지수 함수(exponential function)]
 (2.2)
(2.2)[삼각 함수(trigonometric function)]
 (2.3)
(2.3)
 (2.4)
(2.4)
 (3.1)
(3.1)
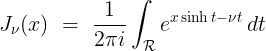 (3.2)
(3.2)
 (3.3)
(3.3)
여기서 사각형 경로상에서
 (2.3)
(2.3)여기서
[거듭제곱 함수(power function)]
 (2.4)
(2.4)여기서
[증명]
감마 함수(gamma function)의 정의를 이용하여 식 (1.1)의 적분을 정리한다.
______________________________
3. 특수 함수의 변환(transform of special functions)
[베셀 함수(Bessel function)] [2]
 (3.1)
(3.1)여기서
[증명]
제1종 베셀 함수를 일반적으로 정의하는 쉴레플리–좀머펠트 적분(Schläfli–Sommerfeld integral)부터 출발한다.
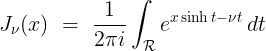 (3.2)
(3.2)여기서
 (3.3)
(3.3) (3.4)
(3.4) (3.5)
(3.5)식 (3.5)에 다시 식 (1.5)를 사용해서 증명을 완성한다.
______________________________
식 (3.5)에서
 (3.6)
(3.6)통상적으로 베셀 함수의 정적분은 구하기 어렵지만, 라플라스 변환 관점의 적분은 조금 수월하다.
4. 길쌈(convolution)

[그림 4.1] 감쇠 지수 함수의 길쌈 예(출처: wikipedia.org)
[정의]
 (4.1)
(4.1)
 (4.2)
(4.2)
[길쌈 정리(convolution theorem)]
 (4.3)
(4.3)
 (4.4)
(4.4)
그러면 식 (4.3)의 첫째식이 증명된다. 식 (4.3)의 둘째식을 위해서는 식 (1.2)를 이용해
 (4.1)
(4.1)길쌈(convolution)은 [그림 4.1]처럼 한 신호를 뒤집어서 다른 신호와 곱하기 때문에, 신호의 응답(response)을 모형화할 때 매우 유용하게 사용된다.
[대수적 성질(algebraic properties)]
 (4.2)
(4.2) (4.3)
(4.3)여기서 둘째식에 나오는 적분은
[증명]
길쌈인 식 (4.1)의 둘째 줄을 식 (1.1)에 대입해서 차례로 정리한다.
 (4.4)
(4.4) (4.5)
(4.5) (4.6)
(4.6)______________________________
[파르세발의 정리(Parseval's theorem)]
 (4.7)
(4.7)[증명]
식 (1.7)을 식 (4.3)의 둘째식에 넣고
______________________________
식 (4.7)을 더 간단히 하기 위해
 (4.8)
(4.8) (4.9)
(4.9) (4.10)
(4.10)
[그림 4.2] 파르세발의 정리를 적용하기 위한 닫힌 경로
라플라스 변환인
 (4.11)
(4.11)여기서 극점(pole)은
[참고문헌]
[1] K. H. Lundberg, H. R. Miller, and D. L. Trumper, "Initial conditions, generalized functions, and the Laplace transform troubles at the origin," IEEE Control Systems Magazine, vol. 27, no. 1, pp. 22–35, Feb. 2007. (방문일 2020-10-09)
[2] "Laplace transform of Bessel function of the first kind," ProofWiki. (방문일 2023-11-12)
[다음 읽을거리]
댓글 없음 :
댓글 쓰기
욕설이나 스팸글은 삭제될 수 있습니다. [전파거북이]는 선플운동의 아름다운 인터넷을 지지합니다.